
# White Papers
A Calibrated Precision GNSS Simulator for Timing Applications
Executive Summary
Testing GNSS receivers specifically for timing applications to determine the accuracy of the receiver’s 1PPS output presents challenges in measuring accuracy to sub nanosecond levels. Using the live sky signals as a test reference has many problems. It has been shown that receiver biases vary at different frequency bands because of varying group delay in the RF circuitry: Biases vary across different GNSS signal types because of the different modulation, various decoding techniques and different correlator sizes, and biases even vary from satellite to satellite for the same signal type because of different transmitter characteristics. Moreover, changing atmospherics over time makes the live sky signals a poor reference for repeatable tests. For all of these reasons, it is desirable to use a GNSS simulator to generate the various constellation signals in a repeatable, reliable fashion.
This white paper will show the results of a project that takes an affordable but fully capable precise GNSS simulator and calibrates its RF output so a receiver’s 1PPS can be measured. The goal is to calibrate the 1PPS output reference output of the simulator relative to the simulator’s RF output to a resolution of 0.1 ns for any particular constellation signal.
The operating bands of interest for the four major GNSS systems are examined: GPS L1, L2 and L5; GLONASS L1 and L2; Galileo E1/E2, E5, E6; and Beidou B1, B2, and B3. The measurements are noted for each operating mode and filter selection possibilities. Also, variations due to temperature changes will be examined.
The results should yield a calibrated simulator for precise timing measurements of GNSS receivers. Although the 1PPS reference pulse output delay will be different for each frequency band, the difference will be known and repeatable, so that a thorough evaluation of GNSS receivers operating with all the major constellations can be made.
Introduction
The RF output of the modulated signal has an on-time point determined by a defined modulation transition. In this particular simulator, the Orolia GSG-64, the equipment outputs a digital 1PPS signal that coincides with the RF output on-time point. The diagram below is a simplified block diagram of the RF circuitry the GSG simulator. GNSS signals created by the simulator are primarily synthesized digitally, and then up-converted to the L-band RF signal as shown.

The GSG-64 contains four boards, each capable of generating frequencies in four frequency bands: L1, L2, L5, and L6. This same digital synthesis and up-conversion is replicated for each band.
Circuit Details
The baseband GNSS signal is digitally created together with a 1PPS signal which is available at the rear panel of the simulator. A single FPGA operating at 192 MHz creates both the baseband and 1PPS signals in perfect alignment (within the tolerances of picoseconds with respect to variations in digital rise times). The 1PPS signal experiences propagation delay from buffering en route from the FPGA to the back panel, but this delay is relatively constant for a given temperature.
The baseband signal experiences a different delay as it is sent through the Digital to Analog Converter (DAC), up- converted to L-band through a mixer, and then passed through a variable attenuator/filter and combiner chain before exiting to the front panel.
The 1PPS signal can be delayed artificially under software control to compensate for all this additional RF delay so that its timing matches the RF output. Via this digital delay adjustment, the 1PPS output pulse is matched to the GNSS signal on-time point to the nearest 5 ns (one period of the 192 MHz digital processing clock). To be used as a timing receiver test device, the delay does not have to be adjusted to zero, it merely needs to be known and constant under particular conditions, so any remaining difference is measured and logged.
Calibration Method
The calibration is done in two separate steps:
- The group delay of the RF circuitry is measured from the RF Output of the Mixer to the RF Output front panel connector. Refer to Figure 1. This is done for all four frequency band centers and for all four circuit boards (16 measurements total).
- The baseband GNSS simulated signal at the IF Input of the Mixer is compared to the 1PPS Output at the rear panel of the simulator on an oscilloscope. For each circuit board and frequency, the 1PPS delay is adjusted under software control.
A special firmware was created to allow the unit to enter an RF calibration mode and generate a special calibration signal. The PRN code and the data messages of a standard GPS signal are set to “0;” however, every 100th data bit is set to “1.” So, at every other PPS you can see the phase change, measured at the IF Input of the Mixer. This makes it easy to see the modulated on-time point on an oscilloscope.
Figure 2 shows the 1PPS alignment with this IF signal. The delay measurement is taken from the 50% rise edge of the 1PPS digital signal to the zero crossing of the 192 MHz IF carrier at the mid-point of the data bit transition. In the example shown in Figure 2, the 1PPS is delayed from the on-time IF transition point by 8.5 ns.
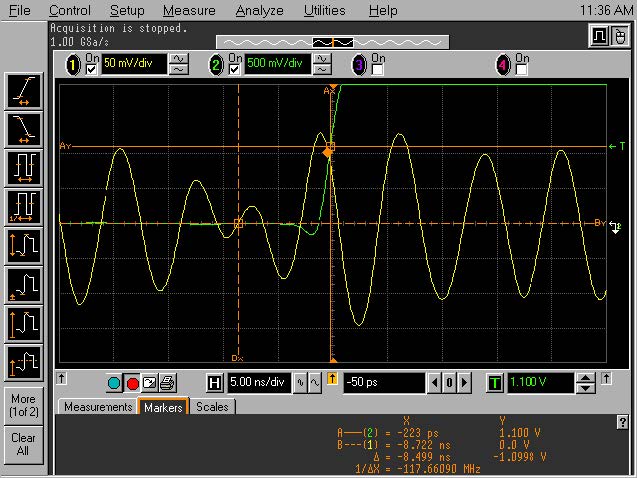
A check is made with a wideband oscilloscope, Teledyne LeCroy 6 GHz. This involves using LNAs to boost the signal to within a visible range. The LNAs will add delay, so they are each calibrated to determine their contribution by removing each one successively and measuring the change in delay. Figure 3 shows the alignment of the 1PPS with the RF signal.
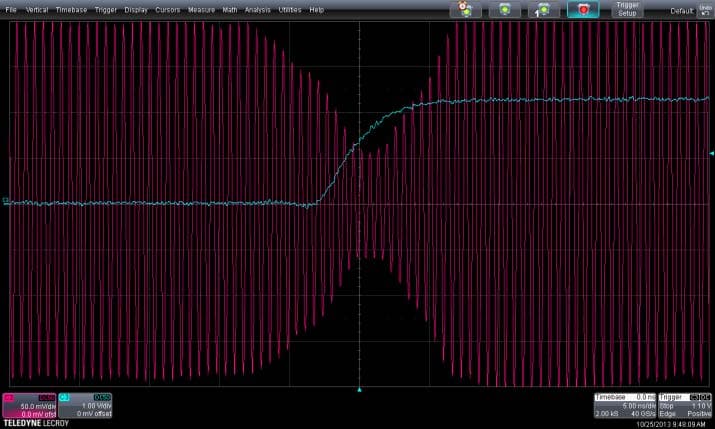
These measurement sets are all taken at 23°C and then selected measurements are repeated at 33°C to determine the variation with temperature.
Measurement Set 1: Mixer to RF output (front panel)
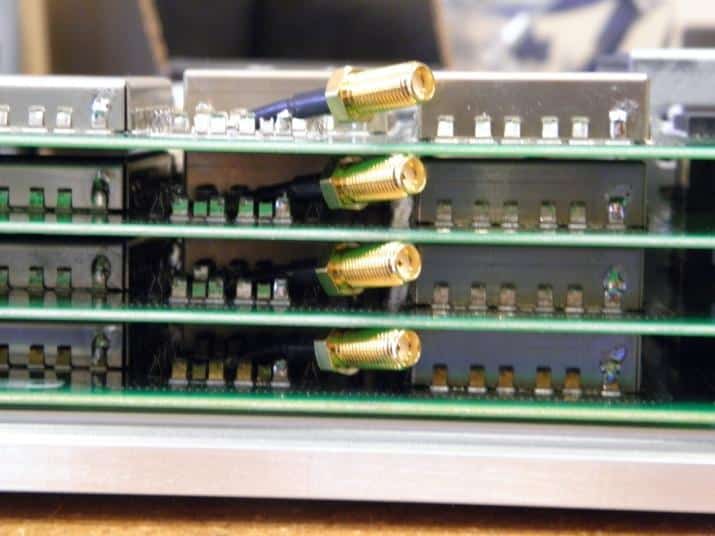
The group delay is measured using a Agilent 4396B Network/Spectrum/Impedance Analyzer with an 87512A Transmission/Reflection Test Kit. The Mixer is removed and an SMA connector is soldered in to provide access to the RF output port as shown in Figure 4. The RF signal from the Analyzer is injected at the SMA connector at the Mixer output. The RF output of the GSG Simulator is connected to the input B of the Analyzer for measurement. After calibrating the cables, the measurements are taken.

Recall that the GSG Simulator is capable of operating in four bands simultaneously because it has four separate boards where the circuitry described is repeated. Any board can operate in any of the four GNSS bands, because they have a software selectable filter bank to switch in for the selected band. There, all four boards are measured at all four center frequencies:
| L1/E1//B1 | 1575.42 MHz |
| L2 | 1227.60 MHz |
| L5/E5/B2 | 1176.45 MHz |
| E6/B3 | 1278.75 MHz |
Sample plots are shown in Figures 6, 7, 8, and 9, with the full results of this measurement given in the detailed test report. The group delay calculation performed by this instrument, shown in the upper right corner of Figure 6 as 8.7848 ns in this example, is calculated across 10% of the frequency span, which is set for 200 MHz, so the group delay is calculated over a 20 MHz bandwidth.

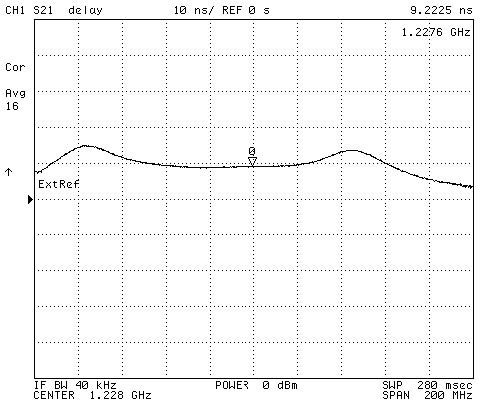
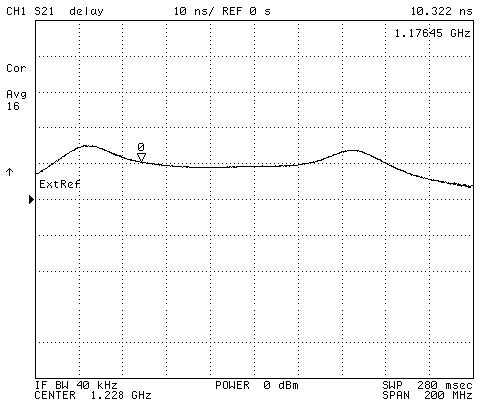
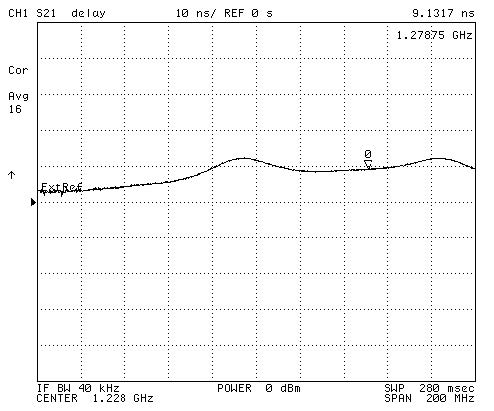
For each of these bands, one can see a nice flatness across the 20 MHz bandwidth which indicates that the phase delay should be fairly constant across the band.
Measurement Set 2: IF to 1PPS
The measurements are made using an HP infinium Oscilloscope 500 MHz, 1 Gsa/s with two Model 1160A 10:1 probes with high frequency ground springs.
The 1PPS is connected to channel 2 on the oscilloscope, using a probe with a ground spring. The IF is measured at the Mixer input test point as shown in Figure 1 with a high frequency probe ground spring.
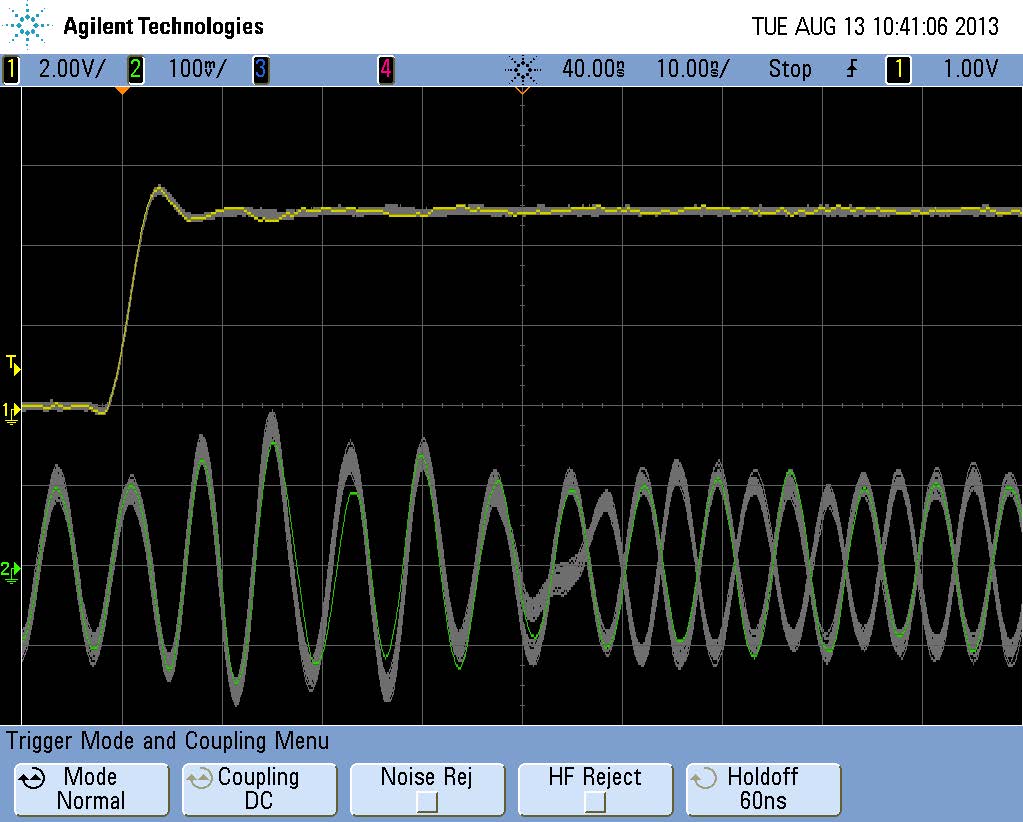
Figure 10 shows the IF on-time point before the delay adjustment is made.
The software offsets are adjusted to compensate for the RF delay and the final results are shown in this table.
| Offset @ IF (ns) | RF Delay (ns) | Offset @ RF (ns) | |
|---|---|---|---|
| Board 0 | |||
| L1 | -7.444 | 8.7848 | 1.3408 |
| L2 | -7.474 | 9.2225 | 1.7485 |
| Board 1 | |||
| L1 | -8.697 | 9.4879 | 0.7909 |
| L2 | -8.99 | 9.9812 | 0.9912 |
| Board 2 | |||
| L1 | -8.697 | 10.173 | 2.936 |
| L2 | -7.15 | 10.147 | 2.997 |
| Board 3 | |||
| L1 | -8.499 | 9.5433 | 1.0443 |
| L2 | -4.632 | 9.6366 | 5.0046 |
Ideally, the values for Board 0 would have been adjusted so the final offset at RF would be very close to zero; however, we found that not every software adjustment to the IF signal could be chosen arbitrarily. There were some values that would not move the IF signal timing. So the closest value was chosen which, in the case of L1 and L2 for Board 0, was -7.4 instead of -8.8 or 9.2. We do not know exactly why this occurs, so further investigations will be made. However, the offset values chosen will yield consistent results.
Accuracy Discussion
An HP Infinium Oscilloscope was used to take the relative time measurement between PPS pulse and RF transition. The delta t accuracy in Real Time Mode is given by the manufacturer as
±[(0.005%)(Δt) + (0.2)(sample period)]
which gives an accuracy of ±200ps for our measurements.
An Agilent 4396B Network/Spectrum/Impedance Analyzer with an 87512A Transmission/Reflection Test Kit was used to measure the group delays. The accuracy of the group delay measurement is the phase accuracy of the instrument divided by the aperture size times 360.
Phase accuracy/(360*aperture(Hz))
The typical phase accuracy for the power levels we are measuring is .3 degree and the aperture was 20MHz (10% of 200MHz span).
Using these values, the calculated accuracy for the measurement is approximately 42ps.
Temperature Variation
The group delay measurements were repeated at a 10oC elevated temperature to measure the variance. The GSG simulator was placed in a temperature-controlled chamber and allowed to stabilize for hours at the higher temperature. The table below shows the results:
| Board 0 | @23C (ns) | @33C (ns) |
|---|---|---|
| L1 | 8.7848 | 8.7595 |
| L2 | 9.2225 | 9.134 |
| L5 | 10.322 | 10.409 |
| L6 | 9.1317 | 8.7654 |
Likewise, the IF to 1PPS oscilloscope measurement was repeated at the elevated temperature and the variation is shown below:
| Board 0 | @23C (ns) | @33C (ns) |
|---|---|---|
| L1 | -2.451 | -2.693 |
For the most part, temperature variation is not a factor and is within our measurement tolerance. With the exception of the L6 band, the RF group delay remained within 0.1 ns. The digital to IF subsection measurement changed 0.25 ns, however, this was for a very large temperature change. For a laboratory instrument that is used in a controlled environment, 10°C is an unrealistic delta. So for more typical laboratory conditions of a couple of degrees of temperature variation, we predict the delay variations will remain within the 0.1 ns range.
Conclusion
By using a two-step measurement process, it is possible to accurately measure and adjust for the offset between the RF and the 1PPS signal in the GSG-64 simulator.
The IF to RF Output group delay measurements are taken and used to adjust the 1PPS output pulse to match the RF output on time point.
Since the offsets are known and repeatable between power cycles, this calibrated simulator can be used to measure and calibrate GPS receivers.
Further investigation is being done to determine if the offset can be adjusted to less than .1ns across all boards and bands.
Acknowledgements
The authors would like to acknowledge the Obseratoire de Paris, LNE-SYRTE, and Dr. Pierre Uhrich and his team for sponsorship of some of this work.
References
[1] Misra and Enge, Global Positioning System: Signals, Measurements, and Performance, 2nd Ed.
[2] Matsakis, D., Mitchell, S., Powers, E., “Satellite Bias Corrections in Geodetic GPS Receivers,” Proceedings of the ION 2013 Pacific PNT Meeting, Honolulu, Hawaii,
April 2013, pp. 577-583
[3] Thölert, S., Grunert, U., Denks, H., “Absolute
Calibration of Time Receivers with DLR’s GPS/Galileo HW Simulator”, 39th Annual Precise Time and Time Interval (PTTI) Meeting
[4] Kalliomaki, K., Mannermaa, J., Mansten, T., “Applicability of Coaxial Cables at Picosecond Rnage Timing,”, Proceedings of the 20th ERTF, 2006, pp. 499 – 502
[5] Plumb, J., Larson, K., White, J., Powers, E., “Absolute Calibration of a Geodetic time Transfer System”, Journal of Letex Class Files, Vol 1, No. 11, Nov 2002
[6] Kaplan and Hegarty, Understanding GPS: Principles and Applications, , 2nd Ed.
[7] IS-GPS-200G, GPS Directorate Systems Engineering & Integration Interface Specification, Navstar GPS Space Sement/Navigation User Interfaces


